Problem C. 1814. (May 2024)

C. 1814. A circle with radius \(\displaystyle r\) is divided into two parts with a line that has a distance of \(\displaystyle \displaystyle{\frac{r}{\sqrt{2}}}\) from the center of the circle. Find the ratio of the areas of the two parts.

Proposed by András Ringler (Budapest)

(5 pont)

Deadline expired on June 10, 2024.

Sorry, the solution is available only in Hungarian. Google translation

Megoldás. Az \(\displaystyle e\) egyenes és az \(\displaystyle O\) középpont távolsága az \(\displaystyle O\)-ból az egyenesre bocsátott merőleges szakasz hossza. Legyen ennek a merőlegesnek a talppontja \(\displaystyle T\).

\(\displaystyle d(O,e)=|OT|.\)

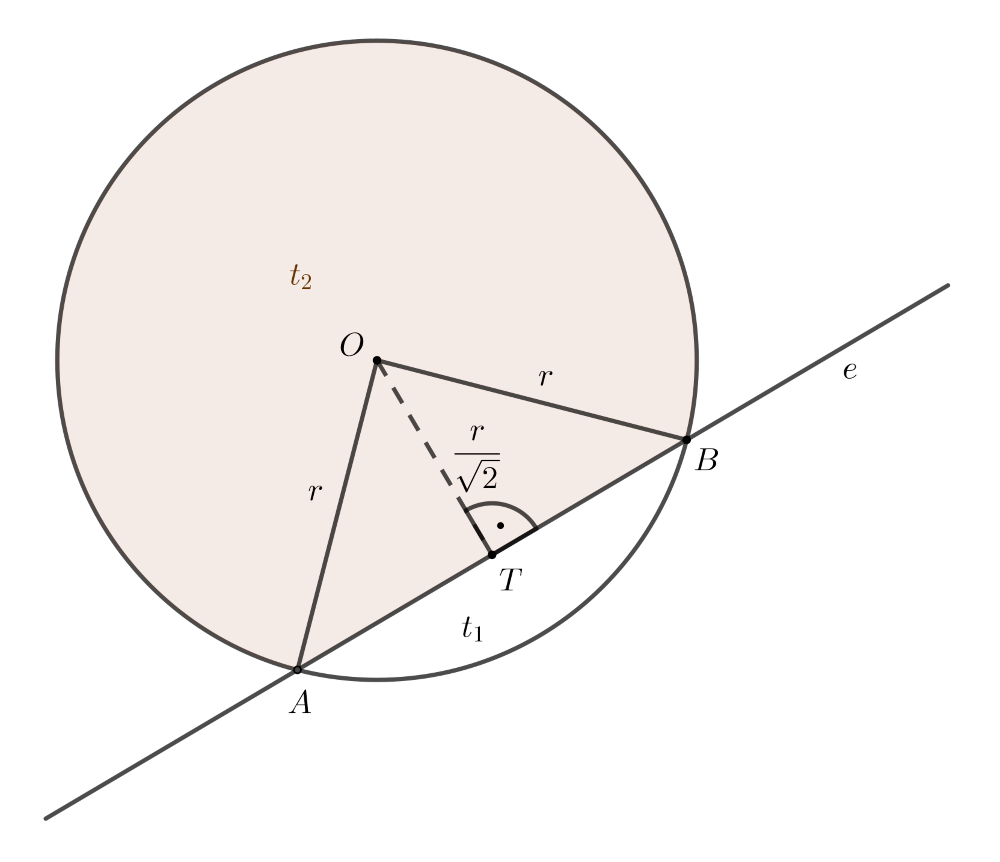
A középpontból a húrra bocsátott merőleges felezi a húrt, ezért az \(\displaystyle AB\) felezőpontja \(\displaystyle T\). Az \(\displaystyle OTB\) háromszög derékszögű, egyik befogója \(\displaystyle \displaystyle{\frac{r}{\sqrt{2}}}\), átfogója \(\displaystyle r\). Erre felírva a Pitagorasz-tételt, azt kapjuk, hogy \(\displaystyle \displaystyle{r^2-\frac{r^2}{2}=TB^2}\), vagyis \(\displaystyle \displaystyle{\frac{r^2}{2}=TB^2}\), tehát \(\displaystyle \displaystyle{TB=\frac{r}{\sqrt{2}}}\). Ez azt jelenti, hogy \(\displaystyle OT=TB\), vagyis az \(\displaystyle OTB\) egyenlő szárú és derékszögű háromszög, így \(\displaystyle TOB\sphericalangle=45^{\circ}\). Az \(\displaystyle OTB\cong OTA\), így \(\displaystyle TOA \sphericalangle=45^{\circ}\), ebből következően \(\displaystyle AOB \sphericalangle =90^{\circ}\). Így az \(\displaystyle A\) és a \(\displaystyle B\) pontok által meghatározott két körcikk területe a kör területének \(\displaystyle \displaystyle{\frac{1}{4}}\)-e, illetve \(\displaystyle \displaystyle{\frac{3}{4}}\)-e.

Az \(\displaystyle e\) egyenes által meghatározott két körszelet területét jelöljük \(\displaystyle t_1\)-gyel és \(\displaystyle t_2\)-vel.

\(\displaystyle t_2=\frac{3}{4}t_{kör}+T_{ABC\triangle}=\frac{3}{4}r^2\pi+\frac{r^2}{2} =r^2\left(\frac{3\pi+2}{4}\right).\)

\(\displaystyle t_1=\frac{1}{4}t_{kör}-T_{ABC\triangle}=\frac{1}{4}r^2\pi-\frac{r^2}{2}=r^2\bigg(\frac{\pi-2}{4}\bigg).\)

A keresett arány:

\(\displaystyle t_1:t_2=\Big(r^2\bigg(\frac{\pi-2}{4}\bigg)\Big):\Big(r^2\bigg(\frac{3\pi+2}{4}\bigg)\Big)=\frac{\pi-2}{4}\cdot\frac{4}{3\pi+2}=\frac{\pi-2}{3\pi+2}\approx0,09992\approx1:10,008.\)

Statistics:

106 students sent a solution.
5 points:	62 students.
4 points:	21 students.
3 points:	6 students.
2 points:	3 students.
1 point:	1 student.
0 point:	1 student.
Not shown because of missing birth date or parental permission:	6 solutions.

Problems in Mathematics of KöMaL, May 2024